|
Home | 18.013A | Chapter 17 |
||
|
|
||
The product rule applied to the curl takes the form
![]()
(This is an immediate consequence of the ordinary one dimensional product rule and the linearity of all our products. When the derivatives here act on f they form the first term here, and when they act on v the produce the second term. The weird star here denotes ordinary multiplication.)
The approach of the last section for reducing computation of divergence in spherical coordinates can be used just as well for the computation of the curl.
The approach, you will remember, consists of finding vectors pointing in the right directions with (0) curl, expressing a general vector as a combination of these, and using the product theorem to express the results. With curl (0) one term of the two in the product theorem disappears and we have our formula.
All you need to do it is to find vectors in each appropriate direction with vanishing curl. That is quite easy to do because the gradient of any function will have vanishing curl.
Thus we can take the gradients of ![]() and of
and of  and of
and of ![]() , and these will be vectors
pointing in the right directions, and give us immediately
, and these will be vectors
pointing in the right directions, and give us immediately
![]()
and so we deduce, via the product theorem
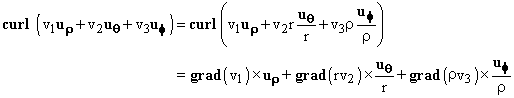
Unfortunately I must admit never ever using this result in any context. So you may safely ignore it, I suppose.
Exercises:
17.5 Find a similar expression for the curl in cylindric coordinates.
17.6 Find the curl of ![]()
|
|