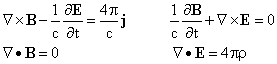 |
|||||
|
|||||
|
|||||
|
|
As we have noted, Ampere's law must be modified in the presence of time dependent currents, because otherwise we would have
By considering what happens when (say alternating) current is passed through
a circuit with a gap, Maxwell realized that consistency requires that there must
be something that contributes to the curl of B in the gap, if there is
such a thing in the circuit. But the gap is empty space to a first approximation;
the only thing present in it is the electric field E that is produced by
the charge density
Exercise 29.1 Take the divergence of both sides here to verify that this equation is consistent with charge conservation. We can put together all the differential equations satisfied by electric and magnetic fields into the following list. These are called "Maxwell's Equation"' although they are much simpler than those he published in 1874. Those had the same content, more or less, but were 20 equations in 20 variables.
The present forms of these equations were obtained by Heaviside who introduced vector notation, divergence and curl. One reason that progress in this area was slow, from Faraday's law of 1831 to Maxwell's equations in 1874 was the difficulty people had in describing multidimensional phenomena and in understanding the equations they wrote, without vector notation. We have here ignored the effects of matter on electric and magnetic fields except in providing electrical current and charge. In fact, matter consists of charge particles with both signs and magnetic dipoles, (like little magnets) and these are affected by electric and magnetic fields. Electric fields cause charge to move in conductors, and polarize non-conductors. By attracting charge of one sign and repelling that of the other they make non-conductors behave like they were full of electric dipoles. As a result, non-conductors modify the effects of fields within them by the fields produced by the polarization and physicists describe these things by defining two kinds of electric fields, D and E and two kinds of magnetic fields, B and H one of which is the field produced by the actual charge distribution, and the other the field including the effects of polarization in matter as well. You will study these things in your physics course. |
|
||||||||||||||||||||||||
.
.
We can see then that a consistent way to change Ampere's law to take into account
varying current is to add a term 
to its left side, to produce:
