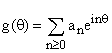|
Suppose we have a function f(z) that is non singular in a unit
circle around the origin. It will then have a power series expansion of the form
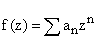
which will have radius of convergence at least 1.
If we look at this function on the unit circle, on which  this series takes the form
this series takes the form  ,
so that if we define ,
so that if we define  we have
we have
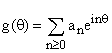
A series of this kind, or alternatively, a series of either of the forms:

is called a Fourier series. The series on the right in each case is periodic
with period 2 .
By adding a constant factor in the arguments of the exponents sines or cosines
you can create Fourier series with other periods as well (replacing for example .
By adding a constant factor in the arguments of the exponents sines or cosines
you can create Fourier series with other periods as well (replacing for example
 you can make the period L.)
you can make the period L.)
The coefficients an here can be determined by use of the integral formulae
of the last section.
Integrating over the unit circle in z we can integrate  to get
to get
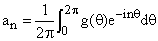
The comparable formulae for bn and cn are
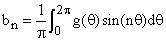
and
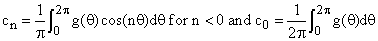
Exercise 30.14 Verify the correctness of these formulae by finding the b's
and c's for 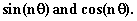
Fourier series representations of functions are very useful because sines,
cosines and exponentials are easy to differentiate. Expressing a function as a
sum of exponential functions is in effect expressing it as a sum of eigenfunctions
of the derivative operator. This is the analog of using a basis in an ordinary
vector space whose members are all eigenvectors of some important linear transformation
on that space.
Obviously Fourier series do not exist for all functions g( ),
and need not for those that are singular in the range of integration. Also, since
cos n ),
and need not for those that are singular in the range of integration. Also, since
cos n is an even function of
is an even function of  for all n values and sin n
for all n values and sin n is an odd function of it, sine and cosine series are, as given here, appropriate
for odd and even functions g respectively.
is an odd function of it, sine and cosine series are, as given here, appropriate
for odd and even functions g respectively.
You can apply the formulae for the coefficients to any function, including a discontinuous
one like a step function, that for example, is 1/2 for angles such that x >
0 and -1/2 otherwise.
All sines and cosines are continuous, so that the partial sums of the Fourier
series for a step function must be continuous. This means they must each be quite
wrong in some interval around a point of discontinuity. Such intervals will typically
shrink as the index of the partial sum increases.
If we integrate f multiplied by its complex conjugate around the unit circle,
and its Fourier Series converges to it almost everywhere, the integral, should
equal the sum of the terms gotten by substituting the series into the integral
for g and its complex conjugate. All cross terms among the series in the latter
give 0 contribution, and we get
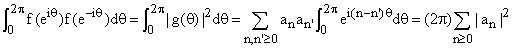
Since the terms in the series on the right here are all positive, its partial
sums must all be less than either of the two integrals on the left.
Mathematicians have devoted an enormous amount of effort to understanding when
Fourier series converge.
Exercises:
30.15 Derive a similar result for the sine and cosine series.
30.16 Calculate the Fourier coefficients of the step function that is 1/2
for  and is -1/2 elsewhere.
and is -1/2 elsewhere.
30.17 Apply the equation just claimed above to this sequence of coefficients.
What do you find?
|

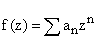
 ,
so that if we define
,
so that if we define