2.25 Problem Sets - Section 5
Problem 5.3: Force on a sluice gate
Previous Problem | Next Problem
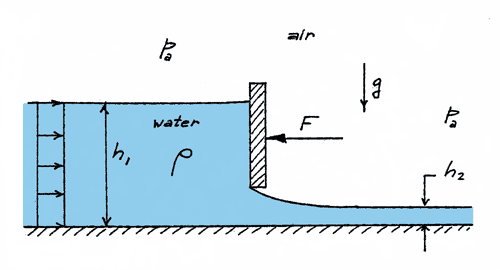
|
Sluice gates are used to regulate water level (or flow rate) in open channels. The figure shows a gate that is adjusted so that the upstream depth is maintained at a depth h1. The density of water is r, and the acceleration of gravity is g. The water flow under the gate may be considered incompressible and inviscid. Suppose the downstream depth is measured as h2, that is, the quantities h1, h2, r, and g are known. (a) Assuming uniform velocities
at the far upstream and downstream stations1 and 2 derive
an expression for the horizontal force F, per unit
breadth, required to hold the gate in place. Check your result
by showing that it gives zero when h1=h2
and the hydrostatic result |
| (b)
Also obtain expressions for the velocities V1and
V2 and the volume flow rate Q per unit
breadth in the stream. Show that as h2 approaches
zero, V2 approaches |
||||