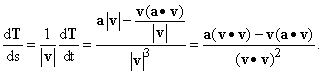» Required Reading » Table of Contents » Chapter 15 15.4 Computation of Curvature
	Previous Section	Next Section

Computation of curvature and the various directions of interest with respect to the curve is quite straightforward, given a parametric representation of the curve, so that we can instruct a spreadsheet to compute everything here along our curve.
The vector 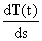, by the chain rule is is the reciprocal of and also can be written as .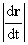
Since T is the unit vector in the direction of .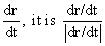
The derivative of this latter expression with respect to t is, by the quotient rule:

We can identify 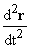with the acceleration of the motion which we denote by a(t).
We need here differentiate |v| with respect to t, which, given that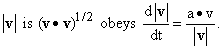

Putting all this together we find:

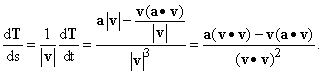
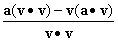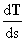This result looks somewhat messy but it actually not so bad. Recall that is the projection of a normal to v. Therefore we have here that is the projection of a normal to v divided by the square of the magnitude of v.
Thus the curvature 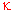, which is the magnitude of this vector, is the component of a normal to v divided by the square of the magnitude of v.

Consider the example of the helix: x  =  cos t, y = sin t, z = t.
We can compute:
Here a and v are perpendicular, so that we get 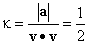for all values of t. The center of curvature is at the reflection of the point on the curve at which we compute it, through the z axis, that is, at the point with coordinates (-cos t, - sin t, t), a distance 2 ( or 1 / ) from (cos t, sin t ,t) in the direction of the projection of a normal to v.

Exercises:

15.1 Show that the curvature of a circle is 1 / r. (This proves that the radius of curvature is 1 /.)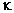

15.2  Find the curvature 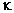position r and center of curvature at t = j / 100 for j = 0 to 100 for the following curve (using a spreadsheet):
x= cos t, y = cos 2t, z = sin 3 t.